Upon initial observation, the fraction 1/281242.5 may seem trivial or even perplexing. However, its true significance emerges when we examine its application across multiple mathematical and practical domains. This seemingly minor fraction encapsulates the concepts of precision and scale, which are essential in disciplines like engineering, finance, and scientific research.
In engineering, for instance, the fraction can represent minute measurements, highlighting the importance of accuracy in the design and construction of structures and machines. In finance, it may be used to express interest rates or ratios, where even the smallest change can have substantial impacts on investment returns. Similarly, in scientific research, such fractions can play a pivotal role in statistical analysis, data normalization, and various calculations that require an acute level of detail.
By exploring the underlying mathematical properties of 1/281242.5, we can appreciate its broader implications. This investigation will not only clarify its relevance but also illustrate how fractions like this contribute significantly to practical applications and enhance our understanding of complex concepts.
Understanding the Fraction 1/281242.5
The fraction 1/281242.5 consists of a numerator of 1 and a denominator of 281242.5, yielding a remarkably small value that signifies a fraction of a whole. This minuscule representation is not just a mathematical curiosity; it holds significant relevance in various practical applications. While the fraction may appear insignificant at first glance, it underscores the importance of precision in numerous fields.
In areas such as engineering, finance, and scientific research, even the smallest variations can lead to considerable outcomes. For instance, in engineering, precise measurements are critical to ensuring the safety and functionality of structures and machinery. In finance, this fraction might be indicative of interest rates or other financial ratios, where minute differences can substantially influence investment strategies and economic forecasting. Furthermore, in scientific studies, 1/281242.5 can be vital for statistical analyses, where accurate calculations and data interpretation are essential for drawing valid conclusions.
The Importance of the Fraction 1/281242.5

Although the fraction 1/281242.5 may initially seem inconsequential, its significance becomes apparent across a range of academic and practical fields. In disciplines such as scientific research, finance, and engineering, the precision represented by this fraction is not only valuable but often essential.
For instance, in scientific research, fractions like 1/281242.5 are critical when analyzing data sets, where even minute differences can lead to different interpretations and conclusions. Similarly, in the finance sector, this level of granularity can influence calculations involving microtransactions, where precise figures can significantly affect overall profit margins or budgeting strategies. In engineering, the fraction could represent tolerances in design specifications, ensuring that machinery operates within safe and efficient parameters.
By recognizing the role of such a tiny fraction, we gain a deeper appreciation for the importance of precision in decision-making processes, particularly in contexts where every number matters. This understanding reinforces the idea that even the smallest values can have profound implications, underscoring the necessity for accuracy in both theoretical calculations and real-world applications.
Practical Applications of 1/281242.5
Real-Where
Fractions such as 1/281242.5 play an essential role in areas that demand exceptional precision. In disciplines including engineering, scientific research, and finance, even the slightest miscalculation can lead to dramatic consequences. For instance, in the design and production of microchips, precise measurements are crucial to ensure optimal functionality. Similarly, in scientific experimentation, where accuracy is paramount, fractions of this nature become indispensable in deriving reliable results. In the financial sector, small decimal variations can accumulate to substantial amounts, especially in scenarios like high-frequency trading or large-scale economic forecasting.
Applications Across Diverse Fields
- Engineering: The fraction 1/281242.5 is particularly relevant when developing intricate schematics or blueprints for machinery that requires high precision. In projects where millimeter accuracy is critical, such small fractions ensure that components fit together seamlessly, thus preventing costly errors and enhancing overall performance.
- Finance: Within the financial realm, fractions like 1/281242.5 can be significant in contexts involving micro-investments or the intricate calculations of exchange rates. Here, even minor decimal adjustments can significantly impact large financial transactions, demonstrating the importance of precision in maintaining accurate accounts.
- Science: In the realm of scientific research, achieving precision down to minute fractions is vital for producing credible results. For example, in fields such as nanotechnology or particle physics, the use of fractions like 1/281242.5 enables researchers to record measurements with the utmost accuracy, facilitating breakthroughs and innovations that rely on detailed data analysis.
By recognizing the importance of small fractions like 1/281242.5, we can appreciate their profound implications in various sectors, highlighting the necessity for meticulous calculations in real-world applications. This understanding not only emphasizes the value of mathematical precision but also illustrates how it influences critical advancements across multiple disciplines.
Mathematical Analysis of 1/281242.5

Evaluating the Value of 1/281242.5
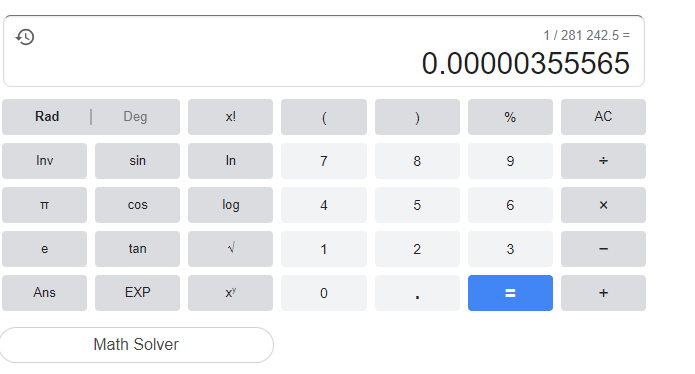
To ascertain the precise value of the fraction 1/281242.5, we conduct a straightforward division operation:
1 ÷ 281242.5 ≈ 0.00000356.
This calculation reveals just how minuscule the fraction 1/281242.5 is. The resulting decimal approximation of 0.00000356 underscores the remarkable level of precision that such small fractions can embody. In numerous fields, including engineering and scientific research, such detailed accuracy is vital. Even a minor deviation in calculations can result in significant variations in outcomes, emphasizing the need for meticulousness in computations.
Bottom
While the fraction 1/281242.5 is already expressed in its simplest form, it’s beneficial to explore the concept of fraction simplification for more complex scenarios. When both the numerator and the denominator share a common factor, one can simplify the fraction by dividing both by their greatest common divisor (GCD). However, since the numerator in this case is 1, it does not have any divisors other than itself, which means that further simplification is not possible.
Understanding the nuances of fractions like 1/281242.5 not only enhances mathematical literacy but also illustrates the fundamental principles of precision and accuracy that are crucial across various disciplines. This awareness allows us to appreciate the role of fractions in practical applications, as they serve as the building blocks for more complex mathematical concepts.
Comparative Analysis of 1/281242.5
With
When juxtaposed with more familiar fractions, such as 1/1000, the fraction 1/281242.5 stands out as significantly smaller, embodying an exceptional degree of precision. While 1/1000 may suffice for many everyday calculations, scenarios demanding heightened accuracy necessitate the use of even smaller fractions like 1/281242.5. Understanding the distinctions between these fractions is essential for professionals in fields where precision is critical, as the choice of fraction can profoundly influence the outcome of calculations and analyses.
Understanding Scale and Impact
The minute scale of 1/281242.5 underscores its vital role in precise measurement applications. In contexts such as extensive datasets, delicate scientific models, or intricate engineering projects, the difference between success and failure can hinge on such small fractions. Even though 1/281242.5 represents a minuscule value, its implications can be substantial in appropriate contexts, impacting everything from research outcomes to engineering efficiencies. Recognizing the significance of such small fractions helps emphasize the necessity for meticulousness in calculations, particularly in high-stakes environments.
story
Origins and Historical Uses
The concept of fractions has been around for thousands of years, with ancient civilizations employing them to depict parts of a whole in various fields, including trade, construction, and early mathematics. Although 1/281242.5 is a contemporary example, the fundamental idea of using small portions to signify precision has historical roots. Early mathematicians and scholars laid the foundation for our current understanding of fractions, evolving from straightforward ratios to complex calculations that incorporate decimals, as illustrated by 1/281242.5.
Evolved
Over the centuries, our methods of calculating and manipulating fractions have undergone remarkable advancements. From the days of manual calculations with pen and paper to the sophisticated computer algorithms of today, the approach to fractions has evolved significantly. Modern technology enables quick and precise calculations involving fractions like 1/281242.5, facilitating heightened accuracy in various sectors, including scientific research, engineering, and financial modeling. This evolution not only enhances our computational capabilities but also broadens the scope of applications for precise measurements in contemporary practices.
Technical Implications of 1/281242.5

Precision and Accuracy
In technical disciplines, the significance of precision cannot be overstated, especially when working with diminutive fractions like 1/281242.5. These small values are critical in ensuring that calculations yield accurate results, as even a minor deviation can result in considerable errors. For instance, in industries such as aerospace manufacturing or medical device production, the integrity of components hinges on precise measurements. In these sectors, a fraction as small as 1/281242.5 can mean the difference between a successful design and catastrophic failure, emphasizing the need for meticulous attention to detail throughout the manufacturing process.
Relevance in Scientific Calculations
In the realm of scientific research, particularly in areas such as quantum physics and chemistry, professionals frequently engage with exceedingly small quantities. Here, fractions like 1/281242.5 often appear within complex equations where maintaining exact figures is essential to uphold the integrity of the results. The inclusion of such precise fractions illustrates the paramount importance of accuracy in scientific endeavors, where even the slightest miscalculation could compromise experimental outcomes and lead to incorrect conclusions.
Visualizing 1/281242.5
Bottom
Challenges and Solutions
Working with small fractions often introduces several challenges, particularly concerning rounding errors and computational accuracy. When handling values such as 1/281242.5, a lack of sufficient precision can lead to cumulative errors that distort the final results. To mitigate these issues, professionals commonly resort to high-precision calculators, specialized software, or sophisticated algorithms designed to accommodate small fractions while preserving accuracy. Furthermore, comprehending the specific requirements of a calculation enables practitioners to select the appropriate level of precision, thereby enhancing the reliability of their outcomes. Through these strategies, the challenges associated with small fractions can be effectively
Educational Insights on 1/281242.5
Teaching 1/281242.5
Incorporating the fraction 1/281242.5 into educational curricula offers a unique opportunity for educators to engage students with the concepts of precision, fractions, and the relevance of small numerical values in practical scenarios. By illustrating how this seemingly insignificant fraction is pivotal in fields such as finance and engineering, instructors can help students connect abstract mathematical principles to real-world applications. For example, discussing how precision in microtransactions or the design of high-tech components hinges on such small fractions can foster a deeper understanding and appreciation among students. This approach not only enhances mathematical skills but also prepares students for future careers in industries where accuracy is paramount.
Tools and Resources for Educators

To effective
It was
As technology advances, our capability to work with and apply small fractions will continue to improve. Innovations in computational methods, data analysis, and machine learning are expected to provide new frameworks for integrating precise values like 1/281242.5 into increasingly complex fields. For example, as industries adopt advanced analytical tools, the importance of accurately processing small fractions will grow, paving the way for developments in sectors such as nanotechnology, pharmaceuticals, and high-frequency trading.
The Role of Technology in Fractional Analysis
The impact of technology on the analysis and application of small fractions cannot be overstated. With the advent of sophisticated software and powerful computing resources, professionals can now handle fractions like 1/281242.5 with unprecedented levels of accuracy. This trend is particularly significant in fields requiring meticulous calculations, where even the tiniest error can have substantial repercussions. As more industries increasingly depend on precise computations, the tools available for fractional analysis will continue to evolve, enhancing our ability to make informed decisions based on accurate data. This convergence of technology and precision will undoubtedly shape the future of various disciplines, reinforcing the need for educational systems to adapt and prepare students for this changing landscape.
Also Read About: 14.86/2/2.5
Summary
The fraction 1/281242.5 may initially appear trivial, but it embodies critical concepts of precision and scale across various fields, including engineering, finance, and scientific research. In engineering, it represents minute measurements essential for safety and functionality, while in finance, it can signify subtle changes in interest rates that significantly impact investment returns. In scientific research, such small fractions are vital for data normalization and accurate statistical analyses.
Despite its minuscule value, 1/281242.5 plays a significant role in practical applications where precision is crucial. For example, in engineering, it ensures the correct fit of components, and in finance, it can influence microtransactions and budgeting strategies. Moreover, in scientific studies, fractions like this are indispensable for deriving reliable results.
Understanding the mathematical properties of 1/281242.5, including its decimal approximation of approximately 0.00000356, reinforces the importance of precision in various calculations. This fraction serves as a reminder of the broader implications of small numerical values, emphasizing the necessity for accuracy in theoretical and real-world applications.
As technology advances, our ability to handle small fractions will improve, enabling even greater precision in fields such as nanotechnology, pharmaceuticals, and high-frequency trading. The role of technology in this domain underscores the importance of educational resources that can help students appreciate the relevance of small fractions in real-world contexts.
FAQS
1. What does the fraction 1/281242.5 represent?
The fraction 1/281242.5 represents a very small value, approximately 0.00000356. It is often used in contexts where precision is essential, such as engineering, finance, and scientific research.
2. Why is precision important in fields like engineering and finance?
Precision is crucial in these fields because even minor inaccuracies can lead to significant errors. In engineering, precise measurements ensure the safety and functionality of structures, while in finance, small variations can affect investment strategies and financial outcomes.
3. How can educators use 1/281242.5 in teaching?
Educators can use this fraction to teach concepts of precision and fractions, illustrating its applications in real-world scenarios such as microtransactions in finance or detailed measurements in engineering.
4. What tools can help students understand small fractions like 1/281242.5?
Interactive simulations, graphical software, and visual aids can enhance students’ understanding of small fractions by providing real-world examples and demonstrating their importance in various applications.
5. What is the significance of technology in handling small fractions?
Advancements in technology allow for high-precision calculations, making it easier to manage small fractions like 1/281242.5 accurately. This is particularly important in industries that rely on meticulous data analysis and calculations.
6. Can 1/281242.5 be simplified further?
No, 1/281242.5 cannot be simplified further because the numerator is 1, which has no divisors other than itself. Therefore, it is already expressed in its simplest form.
7. In what practical applications is 1/281242.5 used?
This fraction is used in applications requiring exceptional precision, such as microchip design in engineering, statistical analyses in scientific research, and calculations in high-frequency trading in finance.
Read Next: 99.99-65-0.23-32.32